Hempel’s Raven Paradox and Inductive Reasoning
In a 1965 work, “Studies in the Logic of Confirmation,” German Philosopher Carl G. Hempel revealed a key paradox in the scientific method as it is generally accepted. He demonstrated the inadequacies in these time-honoured procedures in a stunning and eloquent dissertation. His Raven Paradox challenged the accepted methods of generalisation, falsifiability, and inductive reasoning.
Introduction
Take into account the following to demonstrate what the Paradox of the Ravens is:
(H1) All ravens are black
(H2) All non-black things are not ravens
H2 is the logically equivalent hypothesis to H1, which holds that all non-black items are not ravens. H1 is the claim that all ravens are black. According to conventional first-predicate logic, this is represented as follows:
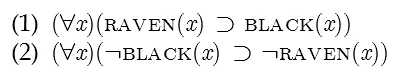
Since (1) and (2) are logically similar, any observation or piece of data that supports either hypothesis must also (equally) support the other. But while it does seem reasonable that observing black ravens should confirm H1, observing a white ball, a red sofa, a yellow shirt, or any non-black non-raven, all of which do confirm H2, also confirm the logically equivalent hypothesis H1 (that “all ravens are black”), which does not seem reasonable.
Two intuitive inductive reasoning principles cause the paradox:
(i) Logically identical assertions can be used interchangeably, and
(ii) Specific examples support the corresponding universal generalisation.
The Origin of the Paradox
Even though the two types of conceptions are very different ontologically, the so-called Paradox of the Ravens results from the erroneous representation of both ontological and logical notions by predicates in conventional first-order predicate logic (FOPL).
A logical representation that treated logical and ontological notions equally, i.e., by considering them as predicates in first-order logic, was the sole cause of the seeming paradox in the so-called Paradox of the Ravens.
Problems with Inductive Reasoning
The Raven Paradox then challenges inductive and deductive reasoning, both of which are essential to the scientific method.
The laws of logic require that a researcher’s conditional statement that all ravens are black has a contrapositive statement. Hence, “Anything that is not black is not a raven,” according to inductive logic. This indicates that every non-black object seen that is not a raven supports the theory in the same way. The universe is filled with an infinite number of non-black items. Therefore, we should feel sorry for the unfortunate statistician who has to study them. Using the analogy, another researcher in a different region of the world might have accidentally only ever seen one raven in their entire life—and it just so happened to be white. They may have concluded that “all ravens are white.” Each non-white thing that is not a raven also strengthens the opposite theory. The Raven Paradox is this.
Falsifiability and Generalization Issue
The Raven Paradox’s initial argument challenges the idea of generalisation.
There might be a few non-black ravens, yet sampling every raven in the world would be almost impossible. Although Hempel wasn’t attempting to remark on the specific science, it’s noteworthy to note that one in every 10,000 raven eggs contains partially or completely albino birds. The majority of albino birds have health issues, are more conspicuous to predators, and may only be a local phenomenon. The likelihood of encountering an albino raven is exceedingly low, and sightings are highly uncommon. Even if there are white birds, a researcher may sample thousands of ravens and not find a single one.
Raven’s Paradox: An end to the scientific method?
The Raven Paradox is a helpful philosophical insight that encourages us to continuously examine and analyse the steps of the accepted scientific procedures. The paradox’s examples are oversimplified and improbable; they are intended only as a test of the limits of the philosophy of science. In practice, the vast majority of the time, Hempel’s thesis has no bearing, and the standard methods of deductive reasoning and experimental design function are just fine. The paradox prevents scientists from concluding that they have proved something beyond a reasonable doubt, which does not diminish research but rather improves it.
Even long-held views that were accepted as unchangeable rules and paradigms might be disproven through time. Testing assumptions and probabilities is the core of science. The most plausible explanation should be adopted if it has a 99% chance of being true. It is extremely unlikely that someone will ever witness a single white raven in their lifetime. But this is not the same as impossible, and that possibility must never be dismissed. To reduce the impacts of the Raven Paradox, all experiments are extensively tested and reviewed before receiving general acceptance. For instance, Newton’s laws were thought to be true until Einstein’s theories disproved them. On the other hand, General Relativity has been replaced by other theories and is not the solution to fundamental physics.
By questioning and changing accepted assumptions and laws, science advances; the development of Chaos Theory is a prime example of “maverick” scientists challenging accepted theories until they could no longer be disregarded. Fractal models gradually became popular, and T-shirts started to bear their prints.
Hence, No theory, no matter how well-established, should be impervious to challenge or discussion, as Hempel’s Raven Paradox serves as a reminder for everyone. Science must adjust and transform as new information is discovered in order to include it.
“Applying Inductive Reasoning: Drawing Parallels Between Observations and Generalizations in Real Life”
We have seen that we frequently experience heartburn after eating spicy food, we can use inductive reasoning to draw the conclusion that spicy food generally causes heartburn. Based on the precise observations we have provided about our personal experiences with spicy food and heartburn, this generalisation has been made.
It’s crucial to remember that not everyone may fall under this generalisation. Some people might be able to eat spicy food without getting heartburn, whilst others might get heartburn from other foods or even from unrelated causes. Therefore, even while inductive reasoning might be effective for drawing generalisations from specific observations, it’s crucial to understand that these generalisations may not always be accurate.
Similarly to this, we can use inductive reasoning to get the conclusion that all ravens are black if we have noticed that every raven we have seen is black. Based on the precise observations we’ve made regarding the colour of the ravens we’ve observed, this generalisation has been made.
Recognising that this generalisation could not apply to all ravens is crucial. There may be variations in colour among different species of ravens, or among ravens from different regions or habitats. In order to draw correct and trustworthy conclusions, it is crucial to combine inductive reasoning with other types of evidence and critical thinking techniques.
Conclusion-
How we interpret the evidence can be strongly impacted by assumptions and background information. We are exposed to a wide variety of fresh ideas and viewpoints, which may contradict some of our preconceived notions and beliefs. The Raven Paradox emphasises the significance of assessing our presumptions and underlying information closely when interpreting data. We can gain a more nuanced understanding of complex topics if we can acknowledge the impact of our own prejudices and opinions
Since inductive reasoning is so common in daily life, being aware of its limitations might help us make better choices. For instance, when we come across new information, we frequently attempt to make sense of it by invoking our prior knowledge and experiences. However, occasionally this can cause us to ignore crucial details and draw hasty conclusions. We can approach new knowledge with a more critical attitude and make more informed decisions if we are aware of the limitations of inductive reasoning.
The Raven Paradox shows that when establishing hypotheses, clarity and specificity are essential. In our coursework, we come across hypothesis testing, which is developing a testable hypothesis and gathering data to confirm or deny it. The Raven Paradox emphasises how critical it is to formulate hypotheses that are specific and explicit in order to make sure they can be tested and refuted.
Inductive reasoning is a key component of the scientific process, but there are also safeguards against its drawbacks. Multiple sources of evidence can help strengthen inductive thinking. We run into difficult problems that demand a thorough study of various points of view and sources of evidence. Multiple sources of evidence, such as professional judgement, data from other research, and historical trends, can increase inductive thinking. We can make a more educated decision by weighing the advantages and disadvantages of various sources of evidence.
The drawbacks of inductive reasoning highlight the value of critical thinking abilities. We are likely to come across a wide range of data and viewpoints that need to be critically assessed. The drawbacks of inductive reasoning emphasise the value of honing critical thinking abilities, such as the capacity to assess evidence, analyse arguments, and identify biases. We can approach new information with a more critical mentality and make more informed decisions by honing these skills.
By Niesshka Barathi
Also Read:
PLAYING THE MARKET: STOCKS OR SLOTS-Deciphering the fine line
Playing the market: Stocks or Slots?Deciphering the Fine line The world of finance, the stock …
Social Echoes: Amplifying Sales through Digital Word-of-Mouth
Social Echoes: Amplifying Sales through Digital Word-of-Mouth It is a truth universally acknowledged that E-Commerce …
Unlocking the Potential: A Comprehensive Analysis of the IMEEEC
Unlocking the Potential: A Comprehensive Analysis of the India-Middle East-Europe Economic Corridor (IMEEEC) Abstract The …
